Welcome to the Onshape forum! Ask questions and join in the discussions about everything Onshape.
First time visiting? Here are some places to start:- Looking for a certain topic? Check out the categories filter or use Search (upper right).
- Need support? Ask a question to our Community Support category.
- Please submit support tickets for bugs but you can request improvements in the Product Feedback category.
- Be respectful, on topic and if you see a problem, Flag it.
If you would like to contact our Community Manager personally, feel free to send a private message or an email.
Moment of Inertia Calculations
 abinand_palanisamy
Member Posts: 4 EDU
abinand_palanisamy
Member Posts: 4 EDU
Hi everyone, I'm new to OnShape, and I have some questions on how the moment of inertia is calculated. My dimensions for this cube is 10 cm for each side length, with a diameter of 8 cm semi-sphere cut out of the top face of the cube. For example, as seen in the image, the moment of inertia along the X-axis is 84.61. Im extremely confused on how this calculation was made, as I thought the formula for moment of inertia was [(Distance from center of mass)^2] * Mass. Wouldn't this give (5^2) * 5.1546029? Is the error within my formula for the moment of inertia?
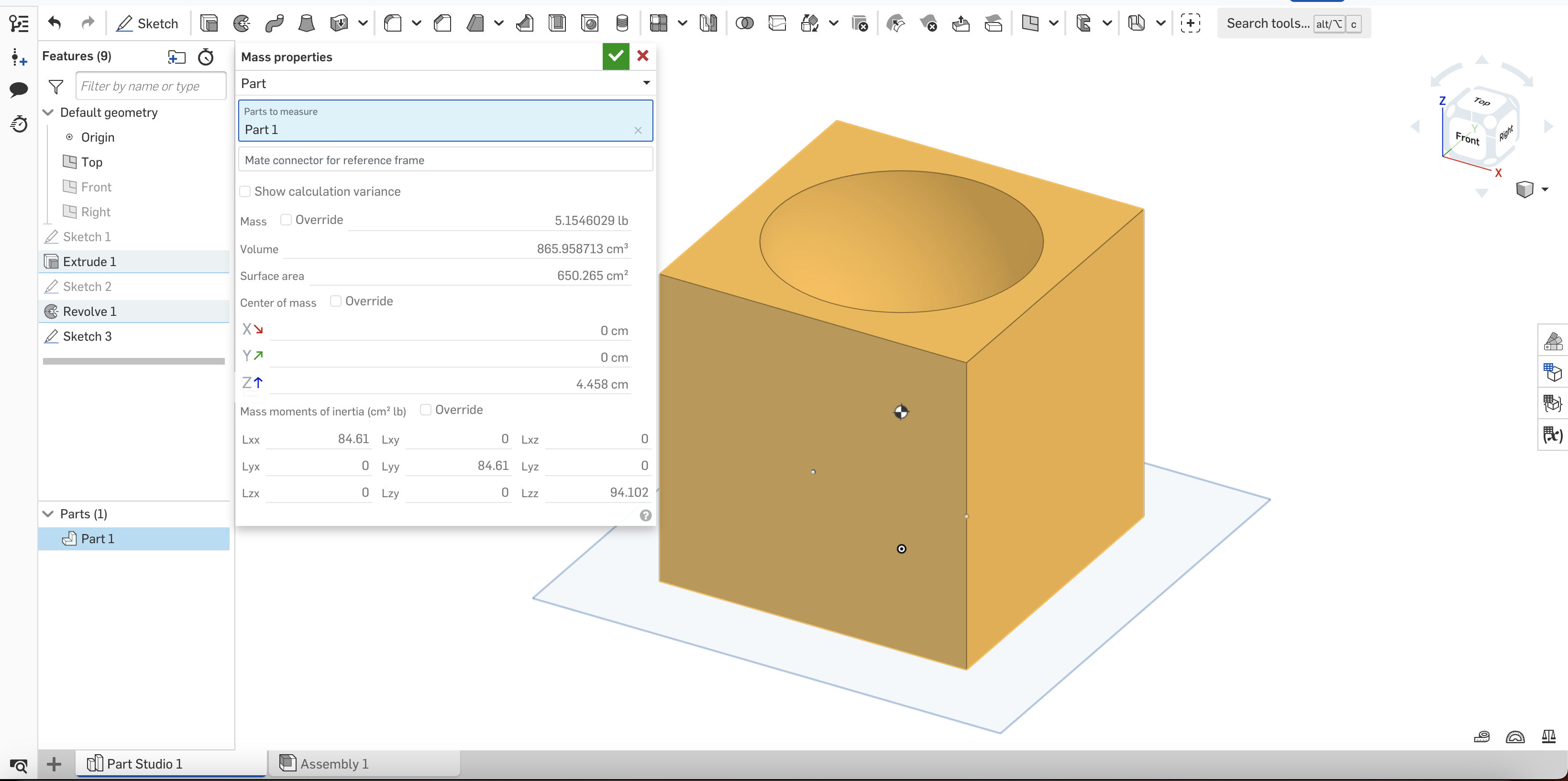

0
Comments
Onshape takes the moment of inertia about the center of mass. So, also as said above, it's easy to verifiy this using simple shapes like cubes, spheres, and cylinders. The center of mass of those is easy. For your shape, it will take a few steps.